Let’s see how to calculate the perimeter and area of a square! These are basic mathematical calculations that every student should know and apply when calculating the perimeter and area of more complex geometric shapes!
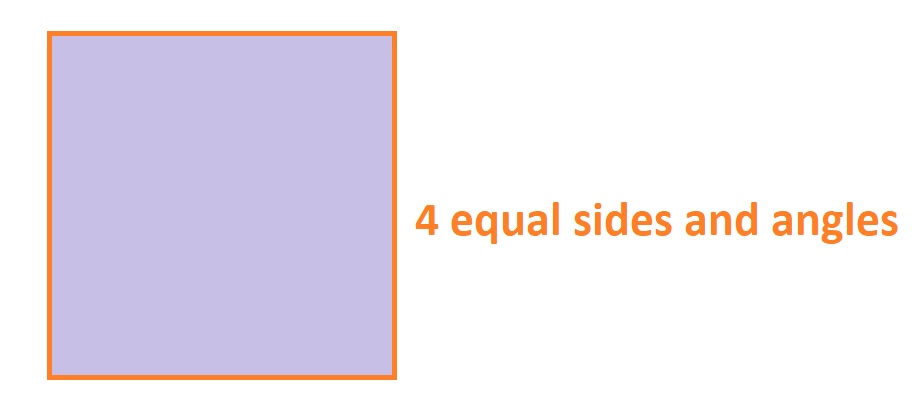
To begin with, let’s remember what a square is! A square is a geometric figure – a 2D shape that has:
- 4 equal sides
- 4 equal angles (4 angles of 90 degrees – right angles)
- 2×2 parallel opposite sides
The square is a geometric figure that children recognize the most when comparing different geometric figures. Even writing notebooks often have lines that are in the shape of squares! This 2D shape has four axes of symmetry. See below in the picture what a square looks like!
In the following text, we will present you with the formulas for calculating the perimeter and area of a square! You must memorize those formulas so that you can always determine the perimeter and area of a square in the future without any problem regardless of the physical characteristics of the particular square!
Formulas For Perimeter And Area Of A Square
See the formulas for calculating the perimeter and area of a square:
Formula for the perimeter of a square:
L= 4 x a
In the formula above, L denotes the perimeter, while lowercase a denotes the length of the side of the square.
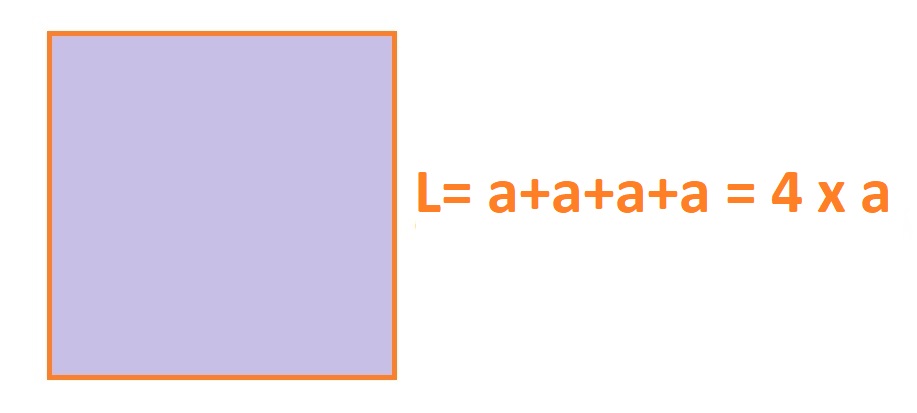
To calculate the perimeter of a square, it is enough to multiply the length of its side by the number 4 (because the square has four equal sides)!
Formula for the area of a square:
P= a x a
In the formula above, P represents the area, while the small a again represents the length of the side of this geometric figure!
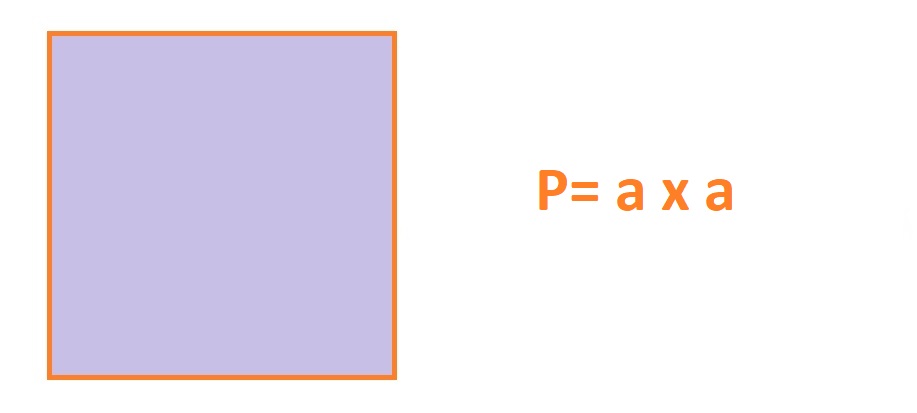
To calculate the area of a square, it is enough to multiply the length of its side by itself!
In the continuation of the text, below, you can see how the mathematical formulas are used to calculate the perimeter and area of a square!
Examples Of Calculating The Perimeter
In this section you can see two examples of calculating the perimeter of a square!
Example 1: Calculate the perimeter of a square that has a side length of 8cm!
Instead of a in the formula for the perimeter of a square, we substitute 8cm. It is important to use the measurement unit cm during the exchange, which will help you to be sure which length measure you should use. The expression after substitution looks like this:
L= 4 x 8cm
For the perimeter of the square from example number one we get that it has the value:
L= 32cm
Example 2: Calculate the perimeter of a square that has a side length of 12hm!
Instead of a in the formula for the perimeter of a square, we substitute 12hm. The expression after substitution looks like this:
L= 4 x 12hm
For the perimeter of the square from example number two, we get that it has a value:
L= 48hm
Examples Of Calculating The Area
In this section you can see two examples of calculating the area of a square!
Example 3: Calculate the area of a square that has a side length of 8cm!
In the place of a in the formula for the area of a square, we substitute 8cm. It is important to use the measurement unit cm during the exchange, which will help you to be sure which length measure you should use. In this situation, be careful, because the same unit of measurement does not remain, but you have to represent it with an exponent of 2! The expression after substitution looks like this:
P= 8cm x 8cm
After multiplication, it is obtained that the area of the square from the example with number three is:
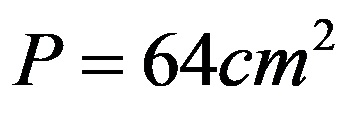
Example 4: Calculate the area of a square that has a side length of 12hm!
In the place of a in the formula for the area of a square, we substitute 12hm. The expression after substitution looks like this:
P= 12hm x 12hcm
After multiplication, it is obtained that the area of the square from the example with number four is:
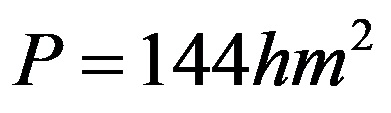
We hope we have helped you learn the formulas and procedure for calculating the perimeter and area of a square. If you are satisfied with our work, share our site with your friends on the social networks that you probably use. Stay connected to our website so you can learn something related to math again in an easy and fun way!




