Math order of operations is the basis of the correct way of calculating a mathematical expression that contains multiple terms related to different mathematical operations! Mathematics is the most precise science that strives to make everything right to perfection!
It is precisely from the above-mentioned directions in mathematics that one must clearly and precisely know according to which rules and order math operations will be carried out and in which way any mathematical problem will be solved. Therefore, this post is intended for all readers to help you in studying this rulebook for math order of operations. Its application will help you solve any problem correctly without doubting the result. In the US the abbreviation for this math order of operations is PEMDAS, while in the UK the abbreviation BODMAS is used for the same rule.
Below we will explain all about using the correct math order of operations!
Math Order Of Operations
If we want to solve any mathematical expression in a correct way, then we should stick to implementing solutions in the following order:
- First we get rid of the auxiliary brackets (if they exist in the expression whose solution we are looking for).
- After getting rid of the parentheses, we solve the exponentiation (if it exists in the expression).
- After finishing with the first two calculations (if any), the math operations of multiplication and division are completed, respecting their position starting from left to right (regardless of whether the division is first and then the multiplication).
- Finally, we continue with addition and subtraction, starting from the left side.
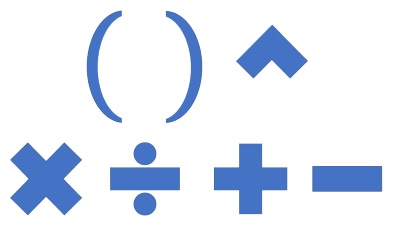
Observing the PEMDAS (BODMAS) rules, we can determine exactly the solution of any mathematical expression. Of course, if any member of the aforementioned does not exist in the current expression we are solving, we skip its corresponding step and continue with the next one!
Below you can see several examples that clearly indicate each step of the math order of operations that should be applied to obtain the corresponding exact solution. Check out the solved examples below!
Example – Math Order Of Operations – Level One
Example 1: Determine the solution of the math expression!
4+(15:3)x2-3=
At the beginning, we get rid of the parentheses. Because 15:3=5, we will write only 5 in place of the parentheses. With this substitution, the following expression is obtained:
4+5×2-3=
Because there is no exponentiation in the expression above, we immediately proceed to the third step of the guide. We continue with multiplication and division. There is only one place in the expression where two numbers are multiplied, so we calculate 5×2=10, and in the place of the product 5×2 we substitute 10 in the next expression that we have to write down! That expression looks like this:
4+10-3=
In the last expression we only have addition and subtraction which are actually the last mathematical operations that we need to calculate in the last step of the guide. That’s why we start from left to right, so first we add 4+10=14, which gives us the expression:
14-3=
To finally subtract the last two numbers, which gives us the correct solution:
14-3=11
Let’s look at another more complicated example with a more difficult mathematical expression in which it is more difficult to apply the mathematical order of operations in the correct way! Continue with example number 2!
Example Level Two
Example 2: Determine the solution of the mathematical expression!
(4+38):6×12+(35:7)x3=
Apparently the expression in the second example has parentheses in two places. That’s why we will get rid of brackets in both places at the same time. We calculate 4+38=42 and 35:7=5, so we write down the obtained results in the place where the brackets are located. After freeing the parentheses, the expression looks like this:
42:6×12+5×3=
There is no exponentiation in the expression, so we keep multiplying and dividing, from left to right, until we neutralize any multiplication or division in the expression! First we calculate what is the leftmost! 42:6=7, so the expression takes the following form:
7×12+5×3=
7×12=84 and 5×3=15. Both products do not have a common member, so we can replace their solutions in one row! The expression after substituting these solutions looks like this:
84+15=
Finally, we have to add the two numbers and get the final correct solution!
84+15=99
We hope that this text and guide helped you to understand what is the correct flow of the math order of operations that must be respected by all of us who in one way or another, directly or indirectly, deal with mathematics! All the best!
PS! Remember, whether we’re talking about PEMDAS or BODMAS, it’s the same instruction for the math order of operations when solving a particular mathematical expression!




